
“Private tutoring and its impact on students' academic achievement, formal schooling, and educational inequality in Korea.” Unpublished doctoral thesis.
CONVERSE GEOMETRY PROBLEMS PROFESSIONAL
Tutors, instructors, experts, educators, and other professionals on the platform are independent contractors, who use their own styles, methods, and materials and create their own lesson plans based upon their experience, professional judgment, and the learners with whom they engage. The resulting theorems are powerful tools you can use to solve many real-world problems. These can be measured, compared, and transformed, and their properties and relationships can be proven using logical deduction. Varsity Tutors connects learners with a variety of experts and professionals. Geometry involves the construction of points, lines, polygons, and three dimensional figures. Varsity Tutors does not have affiliation with universities mentioned on its website. Media outlet trademarks are owned by the respective media outlets and are not affiliated with Varsity Tutors.Īward-Winning claim based on CBS Local and Houston Press awards. Names of standardized tests are owned by the trademark holders and are not affiliated with Varsity Tutors LLC.Ĥ.9/5.0 Satisfaction Rating based upon cumulative historical session ratings through 12/31/20. Since Δ A B C is congruent to Δ P Q R and Δ P Q R is a right triangle, Δ A B C must also be a right triangle. So, the two triangles are congruent by the Side-Side-Side Congruence Property. In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. That is, all the three sides of Δ P Q R are congruent to the three sides of Δ A B C. The sum of the areas of the two squares on the legs ( a and b) equals the area of the square on the hypotenuse ( c ). Since P Q and A B are lengths of sides, we can take positive square roots. P R = a, Q R = b and ∠ R is a right angle.īy the Pythagorean Theorem, ( P Q ) 2 = a 2 + b 2.īut we know that a 2 + b 2 = c 2 and a 2 + b 2 = c 2 and c = A B. Let us assume that c 2 = a 2 + b 2 in Δ A B C and the triangle is not a right triangle. If m is an odd number, then it is a prime number. If m is not an odd number, then it is not a prime number. If m is a prime number, then it is an odd number. Suppose m is a fixed but unspecified whole number that is greater than 2. One of the problems uses coordinate geometry. If the square of the length of the longest side of a triangle is equal to the sum of the squares of the other two sides, then the triangle is a right triangle. 1: Related Conditionals are not All Equivalent. Converse Gridded Response Solve each exercise and enter your answer on the grid provided. In other words, if p q is true and q p is true, then p q (said p if and only if q ). When the original statement and converse are both true then the statement is a biconditional statement.
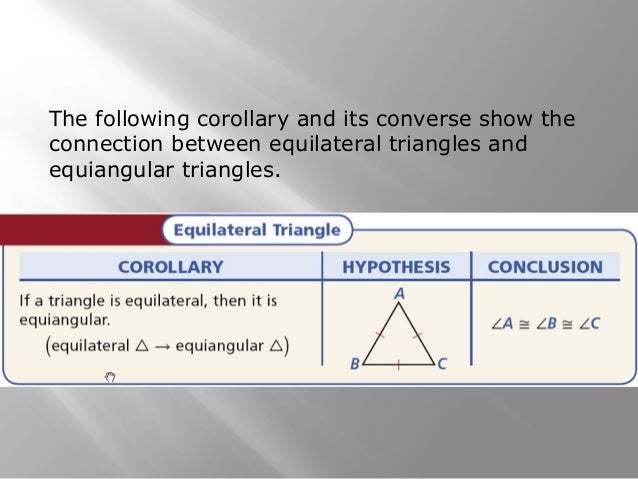

The converse and inverse may or may not be true. The converse of the Pythagorean Theorem is: The contrapositive is logically equivalent to the original statement.
We assume you're familiar with the Pythagorean Theorem.



 0 kommentar(er)
0 kommentar(er)
